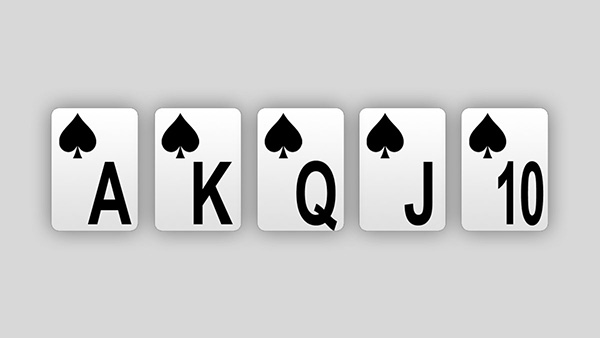
The odds of flopping a straight flush with a premium suited connector such as T9s is 0.02% or 1 in 4,900
Definition of the Straight Flush –
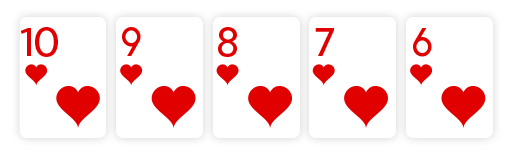
Five cards of consecutive rank, all of the same suit.
Example – 5d6d7d8d9d
The Ten to Ace Straight Flush is the strongest hand in poker and is referred to as the “Royal Flush”.
Odds of Making a Straight Flush on the Flop
Flopping a Straight Flush seldom happens in poker. We specifically need to start out with two suited connected cards for this to be possible.
The odds of flopping a Straight Flush are so unlikely (0.02% or less) that the majority of poker equity calculators don’t even show the precise odds.
We’ll need to do some maths of our own.
Calculation of Straight Flush Odds
Let’s start with a very specific example -
We hold A2s. What are the odds of flopping the Ace to Five Straight Flush?
Why do we choose this example? It’s the easiest one because it provides only one way of making the Straight Flush. The flop has to come down precisely Three, Four, Five of the correct suit.
So, how likely is this?
In order to calculate, we’ll first need to know how many combinations of three cards are possible on the flop.
Basic Combinations and Permutations
Firstly, how many different combinations of three cards can be dealt on the flop? Assuming we care about the order of the three cards (and that our two hole cards are already known), the answer is 117,600 (50 * 49 * 48).
In statistics, this type of calculation is referred to as a permutation and accounts for the order of the flop cards.
Of course, in Hold’em, the order of the cards on the flop doesn’t matter (i.e. a 3,4,5 flop is the same as a 5,3,4 flop, for all intents and purposes). What we are interested in is the number of possible combinations of three cards.
A combination is similar to a permutation but doesn’t account for the order. Since there are 6 possible ways of arranging three cards, we can simply divide our number of permutations (117,600) by 6 to establish the number of possible three-card combinations on the flop.
117,600 / 6 = 19,600 possible combinations of three cards on the flop (given two cards are known)
In other words, there are 19,600 different possible sets of three cards that may fall on the flop given that our two hole cards are already known.
Guess what?
To make the exact Straight Flush in question, only one of these 19,600 combinations will do the job.
Armed with that information, we can now establish a range of different probabilities.
Odds of flopping the Straight Flush with A2s = 1/19,600 = 0.00005 or roughly 0.005%
That’s an insanely small likelihood!
Thankfully, the odds with different types of starting hands are usually a little better.
It all depends on the number of different combinations of three cards that provide a Straight Flush.
For example, think about T9s.
How many different ways are there to make a Straight Flush with 9Ts?
Ways of making a Straight Flush with T9s
JQK
QJ8
J87
678
So that’s four different ways. We are hence four times as likely to make a Straight Flush with 9Ts as we are to make a Straight Flush with A2s.
Odds of flopping the Straight Flush with 9Ts = 4/19,600 = 0.0002 or roughly 0.02%
Ways of making a Straight Flush with T8s
QJ9
J79
679
Odds of flopping the Straight Flush with T8s = 3/19,600 = 0.00015 or roughly 0.015%
Ways of making a Straight Flush with T7s
J89
689
Odds of flopping the Straight Flush with T7s = 2/19,600 = 0.0001 or roughly 0.01%
Only suited connectors (or gappers) can make Straight Flushes on the flop. All other holdings such as pocket-pairs and off-suit combos can never flop a Straight Flush.
We are, naturally, more likely to flop a Straight Flush draw as opposed to the Straight Flush itself. To see examples of calculating the odds of hitting a Straight Flush draw on the flop, check out the 888poker article on Royal Flush odds in poker.